Crustal structure of the Volgo–Uralian subcraton revealed by inverse and forward gravity modelling

Volgo–Uralia is a Neoarchaean easternmost part of the East European craton. Recent seismic studies of the Volgo–Uralian region provided new insights into the crustal structure of this area. In this study, we combine satellite gravity and seismic data in a common workflow to perform a complex study of Volgo–Uralian crustal structure, which is useful for further basin analysis of the area. In this light, a new crustal model of the Volgo–Uralian subcraton is presented from a step-wise approach: (1) inverse gravity modelling followed by (2) 3D forward gravity modelling.

First, inversion of the satellite gravity gradient data was applied to determine the Moho depth for the area. Density contrasts between crust and mantle were varied laterally according to the tectonic units present in the region, and the model is constrained by the available active seismic data.

The Moho discontinuity obtained from the gravity inversion was consequently modified and complemented in order to define a complete 3D crustal model by adding information on the sedimentary cover, upper crust, lower crust, and lithospheric mantle layers in the process of forward gravity modelling, where both seismic and gravity constraints were respected. The obtained model shows crustal thickness variations from 32 to more than 55 km in certain areas. The thinnest crust with a thickness below 40 km is found beneath the Precaspian basin, which is covered by a thick sedimentary layer. The thickest crust is located underneath the Ural Mountains as well as in the centre of the Volgo–Uralian subcraton. In both areas the crustal thickness exceeds 50 km. At the same time, initial forward gravity modelling has shown a gravity misfit of ca. 95 mGal between the measured Bouguer gravity anomaly and the forward calculated gravity field in the central area of the Volgo–Uralian subcraton. This misfit was interpreted and modelled as a high-density lower crust, which possibly represents underplated material.

Our preferred crustal model of the Volgo–Uralian subcraton respects the gravity and seismic constraints and reflects the main geological features of the region with Moho thickening in the cratons and under the Ural Mountains and thinning along the Palaeoproterozoic rifts, Precaspian sedimentary basin, and Pre-Urals foredeep.

1 Introduction

Crustal thickness and thicknesses of individual layers of the Earth's crust play a determining role in estimating the thermal field due to the relative abundance of the radioactive heat-producing elements in the crust (Beardsmore and Cull, 2001; Bouman et al., 2015; Hantschel and Kauerauf, 2009). This fact is particularly important in the case of the Volgo–Uralian subcraton as it is located underneath the Volga–Ural oil- and gas-bearing province with several giant oil fields, where the maturity of the organic-rich rocks is considered to be tightly related to the temperature distribution in the crust (Khasanov et al., 2016; Khristoforova et al., 2008). Therefore, having the knowledge of the Volgo–Uralian crustal structure is the first major step into further basin analysis of the area.

Volgo–Uralia is a large easternmost segment of the East European craton (EEC). It has been regarded as a separate subcraton along with Sarmatia and Fennoscandia since the end of the 20th century (Gorbatschev and Bogdanova, 1993). The Volgo–Uralian part of the EEC is mostly embedded in the East European (Russian) platform, and like the rest of the platform, it does not show any significant topographic variations. It represents a flat area with absolute relief heights ranging from 50 to 250 m for most of the territory. Despite the unremarkable topography of Volgo–Uralia, the same does not hold for its lithospheric structure. Different crustal layers of the subcraton show thickness variations in the order of several tens of kilometres (Artemieva, 2007; Artemieva and Thybo, 2013; Mints et al., 2015).

Several recent crustal models which encompass Volgo–Uralia are based for the most part on regional seismic investigations (Artemieva and Thybo, 2013; Mints et al., 2015). Nevertheless, the gravitational field can also be an essential constraint for the Moho depth especially on the areas devoid of seismic data or with moderate seismic coverage (e.g. Aitken et al., 2013; Steffen et al., 2017). Nowadays, due to the advent of satellite gravimetry, it is possible to obtain gravity field maps with uniform coverage for almost any desired territory of the Earth with a resolution sufficient for regional Moho depth investigation (Bouman et al., 2015).

In this paper, we present a novel model of the Volgo–Uralian subcraton's crustal structure based on inverse and forward 3D gravity modelling with seismic constraints. The main objective of the study is to build a regional crustal model of Volgo–Uralia which in turn can serve as a basis for the further geothermal modelling of the area. In this paper, Sect. 2 is devoted to a brief overview of the tectonic setting and history of the region. Section 3 gives an outlook on the methods and datasets that were used in the study. All the used datasets are outlined in Sect. 3.1. Applied gravity inversion methods are discussed in Sect. 3.2, which is followed by Sect. 3.3, where the process of forward gravity modelling is described. The main results of the inverse and forward gravity modelling as well as the final crustal model of Volgo–Uralia and its comparison to other existing models are presented and discussed in Sect. 4.

2 Tectonic setting of the Volgo–Uralian subcraton

The present-day tectonic setting of the Volgo–Uralian region has formed throughout the assembly of the EEC. It started with the collision of Volgo–Uralia and Sarmatia at 2.1–2.05 Ga which led to the creation of a megacontinent Volgo–Sarmatia with Volga–Don collisional orogen developed on the junction zone between the two segments (Bogdanova et al., 2016). Later, during Meso- and Neoproterozoic times, the Pachelma aulacogen was formed along the Volgo–Uralia–Sarmatia border, which, in combination with the Precaspian sedimentary basin, now delineates the south-western border of the Volgo–Uralian subcraton (Fig. 1). After several hundred million years, at 1.8 Ga, the collision between Volgo–Sarmatia and Fennoscandia commenced. It ended during the formation of the Rodinia supercontinent at 1.0 Ga. The suture intervening Fennoscandia and Volgo–Sarmatia was the place of Central Russian orogeny growth which then was reworked into Central Russian and Volyn–Orcha rifts. At present, the Central Russia rift system represents the north-western border of the Volgo–Uralian subcraton (Bogdanova et al., 2016). On the east, Volgo–Uralia is separated from the West Siberian basin by the young Late Palaeozoic Uralide orogen and Late Proterozoic Timanide orogen (Artemieva, 2007).

Figure 1 Main tectonic elements of Volgo–Uralian subcraton (redrawn after Bogdanova et al., 2016).

In contrast to Sarmatian and Fennoscandian segments of the EEC, Volgo–Uralia, except for the Taratash complex, is completely covered by Neoproterozoic to Phanerozoic sediments which prevent direct studies of the rocks from the outcrops. Nonetheless, extensive drilling activity due to the high hydrocarbon potential of the region has provided numerous core samples of the basement which are telling the composition and the age of the Volgo–Uralian segment (e.g. Bogdanova et al., 2010).

For the most part, Volgo–Uralia is comprised of Archaean continental crust, which is concentrated in large blocks surrounded by Palaeoproterozoic mobile belts. The two most prominent blocks of Archaean crust are the Meso- to Neoarchaean Tokmovo megablock and Palaeo- to Neoarchaean Middle Volga megablock, which in the literature are often associated with the so-called “ovoid” patterns of geophysical anomalies (Bogdanova et al., 2016; Mints et al., 2010). These blocks are dismembered by Elabuga and Chusovaya deformation belts and correspond to relative crystalline basement highs. The sedimentary thickness of the Archaean part of Volgo–Uralia rarely exceeds 2 km. Local increases in thicknesses of sedimentary cover are observed in Palaeoproterozoic aulacogenic, and graben-like structures and can reach 5–10 km (Shargorodskiy et al., 2004). A regional trend of a considerable increase of sedimentary cover thickness is observed towards the Ural Mountains in the system of Kama–Belsk rifts (Fig. S1 in the Supplement). Especially thick sedimentary sequences are located to the south of the Volgo–Uralian subcraton where it reaches the Precaspian depression. There sediments have accumulated in successions with a thickness of more than 20 km (Artemieva and Thybo, 2013).

Stratigraphically, the oldest sediments that have accumulated on the Volgo–Uralian territory are of the late Proterozoic age. They can be found sporadically in deep aulacogenic structures within the cratonic area or the deepest zones of large depressions like the Precaspian basin (Postnikov, 2002; Muslimov et al., 2007). The most extensive sedimentation started in the Middle Devonian and was present throughout the Carboniferous and Early Permian periods. Mesozoic sequences were developed in the north-western and southern peripheries of Volgo–Uralia. Cenozoic sediments are present only in the southern part of the region (Postnikov, 2002). Active Palaeozoic sedimentation in concert with subsequent vertical tectonic movements during the Alpine orogenic phase led to the formation of large arch-like structures surrounded by various troughs. These structures now shape the geometry of sedimentary successions on the Volgo–Uralian subcraton (Bogdanova, 1986; Muslimov et al., 2007). Some of the most prominent structures are the North and South Tatar arches (Fig. S2 in the Supplement). These are the gently sloping uplifts of the sedimentary sequences which started to form in the Middle-to-Late Devonian (Bogdanova, 1986). During the Palaeozoic time, they were mostly the place for marine carbonate sedimentation which was rarely interrupted by upward tectonic movements with marine regression and terrigenous rocks formation. Both North and South Tatar arches are on the relatively uplifted crystalline basement and are reflected in the topography (Burov et al., 2003). Moreover, the South Tatar arch, despite being similar to its northern counterpart, is a very peculiar structure itself. Not only it is an outstanding petroleum-bearing region that holds the giant Romashkino oil field, but it is also a place of active fluid circulation in-between the sediments and the crystalline crust as indicated by Plotnikova (2008). That there is fluid circulation is supported by several phenomena, one of which is decreased density of oil within the South Tatar arch that could result from outgassing of the crystalline basement (Plotnikova, 2008). Overall, it can be said that sedimentary structures of Volgo–Uralia are linked to the fault block structure of the crystalline basement which is partly inherited from the old Precambrian crustal complexes (Postnikov, 2002).

In terms of the crustal structure, Volgo–Uralia is generally a region of thick and dense crust principally in its Archaean part (Bogdanova et al., 2016). Locally, crustal thickness can reach depths up to 60 km in the centre of the craton. The evidence of such thick crust in Volgo–Uralia is found in the recent seismic survey of Tatarstan, where several crustal roots plunging to depths of more than 55 km were disclosed by the TATSEIS-2003 reflection profile (Artemieva and Thybo, 2013; Trofimov, 2006). Relatively shallow Moho was observed seismically within the Central Russian and Pachelma Palaeoproterozoic rifts representing suture zones between individual segments of the EEC. Another region with documented thin crust is the Precaspian sedimentary basin where the crust is thinning down to 32–36 km (Artemieva, 2007). The recent seismic model EUNAseis suggests that Volgo–Uralia has a thick upper crust (with thickness of more than 30 km in some places) which is associated with the above mentioned crustal roots (Artemieva and Thybo, 2013). Earlier findings reveal the correlation between the thicknesses of crustal layers and the tectonic history of the region. That is to say, there is thickening of the upper crust along the Central Russia Palaeoproterozoic rift system and thickening of the lower crust beneath the Archaean blocks of the subcraton (Artemieva, 2007).

3 Data and methods

The work was subdivided into two main steps to build a crustal model of Volgo–Uralia:

1. Gravity field inversion where a preliminary estimate of the Moho depth boundary is obtained (see a detailed description in Sect. 3.2).

2. 3D forward gravity modelling where an extensive crustal model of Volgo–Uralia is built. The model incorporates sedimentary, crustal, lithospheric mantle, and asthenospheric layers along with the previously obtained Moho interface (see a detailed description in Sect. 3.3).

Before the inversion, the gravity data was preprocessed by calculating and subtracting the sedimentary cover effect from the topographically corrected vertical gravity gradient anomaly. The schematic workflow of the study is shown in Fig. 2.

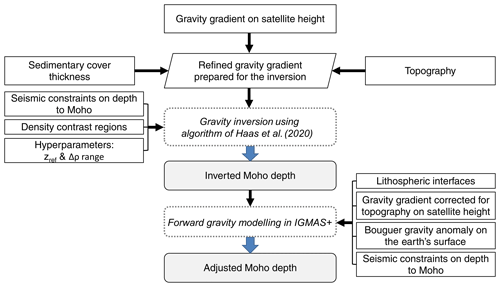
Figure 2 Schematic workflow of the study. The initial step is to prepare the gravity data for the inversion by subtracting the sedimentary cover effect from the topographically corrected vertical gravity gradient. Then it can be followed by a subsequent gravity inversion with laterally variable crust–mantle density contrast (Haas et al., 2020). The inverted Moho depth is incorporated along with other lithospheric interfaces as well as seismic and gravity constraints in IGMAS+ software (Schmidt et al., 2020; Götze and Lahmeyer, 1988). The interfaces are modified to fit the existing gravity and seismic constraints providing the adjusted Moho depth of the region.

3.1 Data description

For successful crustal model construction, four main groups of data were utilized:

• Seismic data used to constrain the Moho during the inverse and forward gravity modelling;

• Gravity data used as a main source of information for gravity inversion and one of the constraints in the forward modelling;

• Structural data, used for inverse and forward gravity modelling;

• Petrophysical data, which were implemented in the forward gravity modelling process.

A summary of the used datasets with their sources is given in Table 1.

Table 1 Summary of datasets used in the modelling.

3.1.1 Seismic data

Seismic estimations of crustal thickness play a crucial role in gravity modelling as they are the main constraint on the crustal structure. We used seismic data within the studied region from the USGS global seismic catalogue (Chulick et al., 2013), which has information on crustal thickness from the main reflection and refraction surveys performed on the Russian platform mostly during the Soviet period. We also added data from recent regional seismic surveys made at the end of the 20th and beginning of the 21st century on the Volgo–Uralian subcraton which were not originally included in the catalogue. These are TATSEIS-2003 geotraverse (Trofimov, 2006) going through the centre of Volgo–Uralia, and URSEIS-95, ESRU, and UWARS profiles which mark the crustal structure on the eastern border of Volgo–Uralia crossing the Ural Mountains (Brown et al., 2002; Thouvenot et al., 1995; Tryggvason et al., 2001). Moho depth estimations from seismic databases used in the study are shown in Fig. 3.

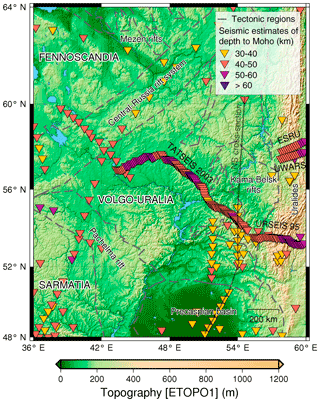
Figure 3 Framework of the studied region with the seismic constraints on Moho depth. Relief is taken from the ETOPO1 model (Amante and Eakins, 2009). Seismic estimates of depth to Moho are used according to USGS seismic catalogue (Chulick et al., 2013), TATSEIS-2003 (Trofimov, 2006), URSEIS-95 (Puchkov, 2010; Tryggvason et al., 2001), ESRU (Brown et al., 2002; Rybalka et al., 2006), and UWARS profiles (Thouvenot et al., 1995).

3.1.2 Gravity data

In the present workflow, the gravity field is the main source of information used for crustal thickness estimation in the area devoid of seismic constraints. It was shown that GOCE gravity gradients on satellite height are sensitive to interfaces with large density contrasts like Moho (Bouman et al., 2015). That is why we utilized topographically corrected GOCE vertical gravity gradient grids on a satellite height of 225 km altitude in the process of gravity inversion (Bouman et al., 2016). In addition, the same topographically corrected GOCE vertical gravity gradient was utilized as a constraint for forward gravity modelling along with the surface simple Bouguer gravity anomaly from the global gravitational model XGM2019e (Zingerle et al., 2019).

3.1.3 Structural data

Several complementary structural datasets were used in the modelling. Surface relief and sedimentary cover thickness are necessary to subtract the gravitational effect of sediments from the topographically corrected vertical gravity gradient field and prepare the gravity data for the inversion (Sect. 3.2). For that purpose, we took ETOPO 1 topographic model (Amante and Eakins, 2009) and sedimentary cover structure inferred from the EUNAseis seismic model for Moho and crustal structure in Europe, Greenland, and the North Atlantic region (Artemieva and Thybo, 2013).

Knowing the structure of the Earth's lithosphere can also be useful in the forward gravity modelling process as the lithosphere–asthenosphere boundary (LAB) is an interface with a density contrast that affects the gravity field. Here, we added the LAB boundary calculated from the concept of thermal isostasy by Artemieva (2019). Being an isothermal boundary, it not only serves as additional density contrast but also provides information about the thermal state of the lithospheric mantle.

3.1.4 Petrophysical data

The main petrophysical parameter which is involved in operations with gravity field is density. The density model used in the study is given in Table 2. Densities of sediments were described by the function of exponential growth of density with depth obtained for the EEC (Artemieva, 2007). Densities of the upper and lower crust were taken based on the seismic estimates of the densities from the CRUST 1.0 model (Laske et al., 2013).

Table 2 Density model used in the study.

^* z – 0.5 of the sedimentary strata middle depth in kilometres.

Upper mantle density was calculated taking into account the contribution of thermal expansion to the density variations in the subcrustal lithosphere assuming that the average lithospheric mantle temperature is a mean temperature between the temperature at the Moho and temperature at the LAB:

$$\begin{array}{}\text{(1)}& {\mathit{\rho }}_{\text{m\hspace{0.17em}\hspace{0.17em}in\hspace{0.17em}\hspace{0.17em}situ}}={\mathit{\rho }}_{\mathrm{m}}\left(\mathrm{1}-\mathit{\alpha }{\displaystyle \frac{T_{\mathrm{M}}+T_{\mathrm{0}}}{\mathrm{2}}}\right),\end{array}$$

where ρ_m – density of the lithospheric mantle at standard conditions, kg m⁻³;

• T_M – temperature at the Moho boundary, ^∘C;

• T₀ – temperature at the LAB, ^∘C;

• α – thermal expansion coefficient, ^∘C⁻¹.

In this study, we consider that the Archaean upper mantle is depleted in mafic components which lowers its density (Kaban et al., 2003). We take the density of the lithospheric mantle of EEC at room conditions of 3340 kg m⁻³, which corresponds to the Palaeoproterozoic–Archaean age (Artemieva, 2007). The temperature at the Moho here is taken as 500 ^∘C which is within the temperature range of Archaean–Palaeoproterozoic crust of EEC according to Artemieva (2007), and LAB temperature as 1400 ^∘C as in our modelling the thermal LAB model of Artemieva (2019) was utilized. It should be noted that normally Moho temperature should depend on crustal thickness (e.g. Mareschal and Jaupart, 2013). Therefore, a constant Moho temperature is a simplification accepted in this study. The thermal expansion coefficient is taken as $\mathrm{3.5}\times {\mathrm{10}}^{-\mathrm{5}}$ ^∘C⁻¹ (Artemieva, 2007, 2019). Using these parameters, we obtained in situ density of the lithospheric mantle as 3230 kg m⁻³.

As the temperature at the Moho boundary does not contribute to the thermal expansion of the asthenosphere, we can slightly modify Eq. (1) to get in situ density of the asthenosphere by taking asthenosphere temperature as equal to LAB temperature:

$$\begin{array}{}\text{(2)}& {\mathit{\rho }}_{\text{a\hspace{0.17em}\hspace{0.17em}in\hspace{0.17em}\hspace{0.17em}situ}}={\mathit{\rho }}_{\mathrm{a}}\left(\mathrm{1}-\mathit{\alpha }{T}_{\mathrm{0}}\right),\end{array}$$

where ρ_a – density of the asthenospheric mantle at standard conditions, which was taken as 3390 kg m⁻³ (Artemieva, 2007).

Asthenosphere density is equal to 3225 kg m⁻³. This leads to a quite moderate density contrast between the lithospheric mantle and the asthenosphere of 5 kg m⁻³, which will not have a big impact on the results of forward gravity modelling.

3.2 Gravity field inversion

3.2.1 Gravity data processing

Gravity field inversion requires initial gravity data to be refined to leave only the gravity signal of interest. In our case, the desired crustal interface is the Moho boundary. In order to obtain the signal that is produced primarily by the Moho undulations, several corrections to the gravity field must be applied. These necessarily would include correction for the latitude, free-air correction, and topographic correction. All the listed corrections are taken into account in the topographically corrected gravity gradient anomaly. We use topographically corrected vertical gravity gradient for the region with 2670 kg m⁻³ rock density and 1030 kg m⁻³ water density.

Another important interface with high-density contrast that causes anomalies on the satellite gravity field of the same wavelength as Moho is the sediments–upper crust boundary (Steffen et al., 2017). Volgo–Uralia, despite not having a large variation in sedimentary thickness in its cratonic part, is neighboured by Pre-Uralian trough and Precaspian basin where sedimentary successions can locally reach 10–20 km thickness (Artemieva and Thybo, 2013; Neprochnov et al., 1970). Therefore, it is essential to subtract the gravity effect of sediments from the topographically corrected gravity gradient to get the refined gravity gradient signal produced by the Moho interface:

$$\begin{array}{}\text{(3)}& G_{\text{REFINED}}=G_{\mathrm{TC}}-G_{\text{SED}},\end{array}$$

where G_REFINED – gravity gradient field prepared for the inversion which reflects mostly the Moho signal, eotvos;

• G_TC – topographically corrected gravity gradient, eotvos;

• G_SED – gravity gradient effect of sediments, eotvos.

As the modelled area is considerably large, we utilized tesseroids to account for the sphericity of the Earth (Uieda et al., 2016). First, the depth of the sediments–upper crust interface was calculated on 1×1 ^∘ mesh using the relief from ETOPO1 and sedimentary thickness from the EUNAseis model. Second, the sedimentary cover was subdivided into a number of tesseroids with lateral dimensions of 1×1 ^∘ and vertical thickness of 1 km. Third, each tesseroid was assigned a certain density depending on its depth. The density–depth relationship for sedimentary cover for the East European platform was taken from Artemieva (2007):

$$\begin{array}{}\text{(4)}& \mathit{\rho }=\mathrm{2430}\cdot z^{\mathrm{0.045}},\end{array}$$

where z – $\mathrm{1}/\mathrm{2}$ of the tesseroid middle depth in km.

Lastly, the gravity effect of sediments was calculated using Tesseroids Python package and it was consequently subtracted from the topographically corrected gravity gradient (Fig. 4).

Figure 4 (a) Vertical gravity gradient corrected for topography on the area of Volgo–Uralian region and (b) refined gravity gradient corrected for both topography and the gravity gradient effect of sediments. Dashed polygons represent tectonic regions used in gravity inversion.

3.2.2 Gravity field inversion with laterally variable density contrast

For the gravity field inversion, we followed the novel approach of Haas et al. (2020) which allows laterally variable crust–mantle density contrasts according to the tectonic regions present in the area of study. This approach solves the inverse problem with the Gauss–Newton algorithm uses second-order Tikhonov regularization to ensure the stability of the solution, and requires two hyperparameters for the inversion: reference Moho depth z_ref and crust–mantle density contrast Δρ with the range of possible values. The algorithm iteratively sets each density contrast from the given range to the predefined tectonic regions keeping spatially constant Δρ within each region. Thus every possible combination of density contrasts' lateral distribution is being checked. Then the combination which gives the smallest RMS error between the Moho depth estimated through the inversion and Moho depth defined at the locations of available seismic measurements is chosen automatically.

Although one can use any gravitational component for the inversion in the abovementioned algorithm, we stuck to the vertical gravity gradient as it is shown to be more sensitive to the Moho undulations than the other components (Bouman et al., 2016). Here, for the purpose of tectonic regionalization, we take the main crustal provinces of Volgo–Uralia from Bogdanova et al. (2016) which include the Archaean cratonic continental crust and Palaeoproterozoic mobile belts. We also distinguished Uralide orogen in a separate tectonic region because of its relatively young age and distinct crustal composition. Another tectonic region that deserves our attention is the Precaspian sedimentary basin. The sedimentary strata in its central part reach 20 km of total thickness and include layers of Permian salt with thickness reaching ca. 4–5 km (Volozh et al., 2003; Brunet et al., 1999), which is unique for the EEC. We distinguished this region as a fourth tectonic unit used for gravity inversion.

For the density contrasts, we chose a range of 350 to 550 kg m⁻³ with a step of 50 kg m⁻³. Such a range was chosen according to the previous findings for the region based on satellite gravity (Eshagh et al., 2016) and seismic studies (Rabbel et al., 2013) which suggested that the density for the region should be between 300 and 600 kg m⁻³. We ran the code 10 times using different reference Moho depths ranging from 41 to 50 km with a 1 km step. The range was taken around the average Moho depth of 45 km suggested by the seismic measurements. Before performing the inversion, we decreased the resolution of the used seismic Moho estimates on the newly digitized profiles to make it similar to the resolution of the USGS seismic catalogue in the study area. The resolution was decreased 4 times: from 10 to 40 km. Finally, the Moho which fitted best to the seismic constraints was selected. All the calculations during the gravity field inversion were performed on a 1×1 ^∘ grid.

3.3 Forward gravity modelling

Gravity inversion was followed by forward gravity modelling which, was done with IGMAS+ software (Götze and Lahmeyer, 1988; Schmidt et al., 2020). IGMAS+ is a geophysical package aimed at 3D numerical modelling, visualization, and interpretation of potential fields. It offers users the ability to combine different sources of data in a common workflow such as seismic constraints, first, and second-order derivatives of gravitational potential, magnetic field data, and other geological and petrophysical information to produce the most accurate model of the earth's interior.

At the beginning of the modelling, the study area was laterally extended by 2500 km to minimize edge effects. This has been done by extending the thicknesses of the modelled layers from the edges of the study area. The dimensions of the modelled study area are 2672 km E–W × 3236 km N–S which means that the taken lateral extension is approximately 95 % of the model's latitudinal and 75 % of its longitudinal dimensions. The vertical depth of the model was chosen to be 300 km in order to include all the interfaces along which the main density contrasts arise starting from the bottom of sediments and finishing with the LAB. The 3D model is constructed by triangulated polyhedrons inbetween 67 vertical cross-sections, which are oriented in the west–east direction. The approximate distance separating the sections is 50 km. IGMAS+ allows to forward calculate the gravity field from the model and cross-compare it with the measured values which allows the model to be enhanced in the places of significant gravity misfit. The full process of forward gravity modelling of the current study can be described in five steps:

1. We imported seismic, structural, and gravity data in IGMAS+: (a) Moho interface derived previously from the gravity inversion, (b) depth of the sediments from EUNAseis model (Artemieva and Thybo, 2013), (c) the depth of the LAB interface obtained from the thermal isostasy method (Artemieva, 2019), (d) Available seismic estimates of the Moho depth from USGS seismic catalogue, TATSEIS, URSEIS, UWARS, and ESRU seismic profiles (Brown et al., 2002; Chulick et al., 2013; Thouvenot et al., 1995; Trofimov, 2006; Tryggvason et al., 2001), (e) Bouguer gravity anomaly from XGM2019e global gravity field model (Zingerle et al., 2019), (f) topographically corrected gravity gradient calculated from the gravity gradient grids (Bouman et al., 2016). Additionally, we subdivided the crust into upper and lower parts with the initially horizontal interface. The densities of all the layers were set to the values according to Table 2. The sedimentary layer was discretized in a number of isometric voxels with a 500 m thickness. It allowed representation of the exponential increase of sediments' densities with depth.

2. We adjusted the structure of gravity inverted Moho boundary where seismic data exposed different depths and when it led to the enhancement of the gravity fit or when the seismic data showed consistently different Moho depths on one of the digitized profiles.

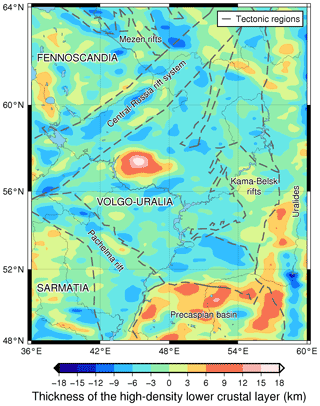
3. We forward calculated gravity and gravity gradient fields from the current model and observed a significant gravity misfit of ca. 95 mGal in the centre of the Volgo–Uralian subcraton. This misfit was attributed to the underplated body with a relatively higher density located in the lower crust (see Sect. 4.2).

4. We estimated mass imbalance (surplus and deficit) in the area by isostatic calculations following the approach of Ebbing (2007) for the Scandinavian mountain chain:

   $$\begin{array}{}\text{(5)}& \begin{array}{rl}{\mathit{\rho }}_{\text{sed}}{D}_{\text{sed}}& +{\mathit{\rho }}_{\mathrm{UC}}{D}_{\mathrm{UC}}+{\mathit{\rho }}_{\mathrm{LC}}{D}_{\mathrm{LC}}+{\mathit{\rho }}_{\mathrm{m}}{D}_{\mathrm{m}}\\ & +{\mathit{\rho }}_a{D}_a-{\sum }_{i=\mathrm{1}}^{\mathrm{5}}{\mathit{\rho }}_{\text{refi}}{D}_{\text{refi}}=\mathrm{\Delta }\mathrm{Load}/g,\end{array}\end{array}$$

   where ρ and D – densities in kg m⁻³ and thicknesses in m of the sedimentary, upper crustal, lower crustal, lithospheric mantle, and asthenospheric layers of the IGMAS+ geological model;

   ρ_refi and D_refi – densities in kg m⁻³ and thicknesses in m of the reference model which are equal to the average values of these parameters used for the corresponding layers in the geological model; ΔLoad – isostatic load reflecting the mass surpluses and deficits in the area, kg m⁻¹s⁻²; g – normal gravity field, m s⁻².

   The density of the underplated body was set to 3100 kg m⁻³ which gives the difference between the lower crustal density and the assumed body of −200 kg m⁻³. We divided the obtained mass imbalance from Eq. (5) by the difference in densities of the lower crust and the assumed underplated body. In this way, we obtained the thickness of the high-density lower crustal layer associated with the underplating.

5. The last step was to modify the geometry of the layers to reach a good fit to the gravity data. Here Moho boundary and upper–lower crust interface were subjected to further modifications. The upper–lower crust interface was modified in order to both provide better gravity fit and resemble the patterns of the bottom of the “felsic-intermediate” crust from the EUNAseis model. The Moho was modified in areas of no seismic constraints to enhance the gravity fit.

4 Results and discussion

As a result, a new crustal model of the Volgo–Uralian subcraton was obtained throughout the gravity field inversion and forward gravity modelling.

4.1 Results of the gravity inversion

In the gravity inversion two hyperparameters, the reference depth and the density contrast, were estimated such that the resulting gravity-inverted Moho showed the minimum RMSE with the seismic Moho depth estimates.

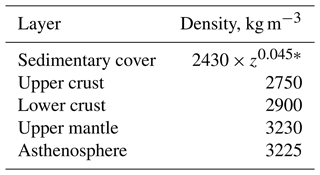
The reference depth which gave the best-fitted Moho to the seismic data was equal to 45 km. Such a relatively deep estimate was obtained due to the fact that TATSEIS-2003 and URSEIS-95 seismic profiles provided a considerable fraction of Moho depths' measurements of more than 50 km. In terms of the density contrast, Archaean cratonic crust and Uralide orogen resulted in a density contrast of 550 kg m⁻³, whereas for the Palaeoproterozoic belts it was equal to 500 and 350 kg m⁻³ for the Precaspian basin (Fig. 5a). These values are close to the previous findings of Eshagh et al. (2016) who used GOCE gravity gradients and determined that crust-mantle density contrast on the territory of Eurasia should be in the range of 400–600 kg m⁻³. At the same time, other seismic-based studies suggest a slightly smaller density contrast around 300–400 kg m⁻³ for the tectonic settings similar to the ones of the modelled region (Bassin et al., 2000; Rabbel et al., 2013). This misfit can arise because gravity-based methods average the crustal and subcrustal densities and express their difference in one signal. Whereas, seismic-based methods restore densities for specific layers in the crust and the lithosphere and give a more targeted look at the contrast in densities between the lower crust and the lithospheric mantle. Our density model used in the forward gravity modelling gives a density contrast of around 330 kg m⁻³ (Table 2) which is closer to the values coming from the seismic-based estimates.

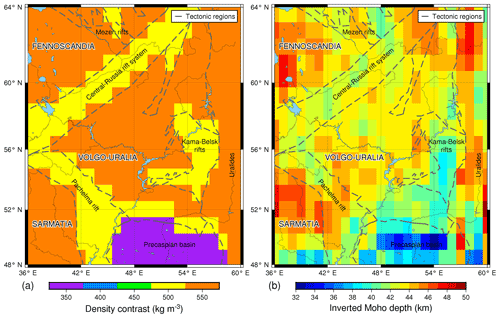
Figure 5 (a) Density contrasts determined by using the algorithm of Haas et al. (2020) and (b) the Moho depth obtained through the gravity field inversion. Reference depth is equal to 45 km, crust-mantle density contrast of 550 kg m⁻³ is assigned to Archaean cratons and Uralide orogen, 500 kg m⁻³ is assigned to Palaeoproterozoic rifts, and 350 kg m⁻³ to Precaspian basin.

The obtained gravity-inverted Moho depth map generally respects the main known structural features of the crust in the region: Moho thickens in the cratons and Uralides, and thins along the Palaeoproterozoic rifts, Pre-Urals foredeep, and Precaspian sedimentary basin (Fig. 5b).

4.2 Results of the forward modelling

The final product of the forward gravity modelling is the IGMAS+ 3D model of the Volgo–Uralian crustal structure. It includes the updated Moho model along with the main crustal interfaces. The constructed IGMAS+ model has a standard deviation of measured and calculated gravity equal to 8.0 mGal which corresponds to the correlation coefficient between the measured and calculated gravity of 0.91 (Fig. 6a–c). For the vertical gravity gradient, the standard deviation is equal to 0.13 eotvos and the correlation coefficient is 0.81 (Fig. 7a–c). This can be considered as an acceptable gravity fit for a regional crustal study (e.g. Sobh et al., 2019). Figure 7 shows a long-wavelength residual in the gravity gradient field. This may potentially point to the lateral density heterogeneity due to compositional change of the crust and lithospheric mantle of Volgo–Uralia which was not taken into account in the current model. The degree of such compositional density variations as well as possible causes of it is discussed in Artemieva (2003). The general look of the IGMAS+ 3D model with the locations of vertical sections is given in Fig. 8.

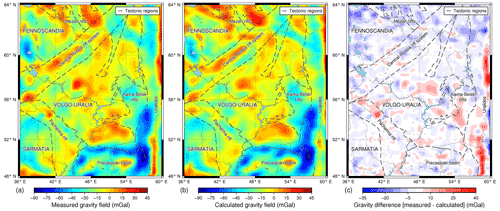
Figure 6 Comparison between measured and calculated gravity fields. (a) XGM2019e Bouguer gravity anomaly (Zingerle et al., 2019). (b) Calculated Bouguer gravity anomaly from IGMAS+ 3D model. (c) The difference between measured and calculated gravity fields.

Figure 7 Comparison between measured and calculated gravity gradient fields. (a) GOCE topographically corrected vertical gravity gradient at 225 km height (Bouman et al., 2016). (b) Calculated topographically corrected vertical gravity gradient field from IGMAS+ 3D model. (c) The difference between measured and calculated gravity gradient fields.

Figure 8 A 3D lithospheric model of Volgo–Uralia developed in IGMAS+ software. It consists of 67 vertical sections which gives a spatial resolution of approximately 50 km. The model includes 5 main layers: sediments (Sed), upper crust (UC), lower crust (LC), lithospheric mantle, asthenosphere, and an additional 6th layer of underplating. The Bouguer gravity anomaly produced by the model is shown on top.

Prior to reaching the aforementioned gravity fit, a considerable misfit of measured and calculated gravity revealed at the initial stage of forward modelling was interpreted and modelled as an underplated material (Sect. 3.3). This misfit arose after fitting the inverted Moho depth to the seismic data in the north-western portion of TATSEIS-2003 seismic profile. The depth difference between the seismic and inverted Moho depth is shown in Fig. 9a. Figure 9b shows the difference between the Moho depth obtained in the forward modelling and the inverted Moho depth. From Fig. 9 one can see that Moho is much deeper in the centre of the Volgo–Uralia in the area of interpreted underplating. This feature is not initially seen on the gravity inverted Moho depth map.

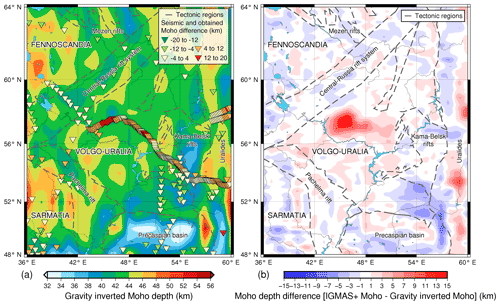
Figure 9 (a) Comparison of the inverted Moho depth and the seismic Moho estimates, where most of the difference is coming from the TATSEIS 2003 seismic profile in the centre of the model. (b) Difference between IGMAS+ and gravity-inverted Moho depths.

The hypothesis of underplating in the area is not new. It was previously suggested by Thybo and Artemieva (2013) and is generally mentioned in the literature (Bogdanova et al., 2016, 2010; Mints et al., 2010). The recovered underplated body appears to be located on the north of the Tokmovo megablock under the Oka block (Fig. 1). This body is defined on a TATSEIS-2003 seismic profile as an acoustically transparent region (Trofimov, 2006). Mints et al. (2010) interpreted this feature as a domain of homogeneous mafic rocks partially metamorphosed into high-density granulites or eclogites at the basis of the so-called Vetluga synform. The isostatic calculations from Eq. (5) also show the high-density body with an average thickness of ca. 10 km which is clearly outlined by the area of isostatic imbalance in the centre of Volgo–Uralia (Fig. 10). Other regions with the major mass deficits are located on the south-east of the map and are related to the Precaspian depression and South Ural orogen. However, they do not correspond to any significant gravity misfit and are produced simply by the high deviation of the sedimentary and crustal thicknesses from the average values on the territory yielding higher values of mass imbalance.

Figure 10 Thickness of the high-density lower crustal layer from the isostatic calculations.

The Moho depth of the developed IGMAS+ model shows a good agreement with seismic constraints (Fig. 11). The mean difference of seismic and modelled Moho is 0.75 km, the standard deviation is 3.31 km. This can be regarded as a satisfactory result as seismic Moho estimates usually are considered to have at least 2 km uncertainty (Ebbing et al., 2012). Therefore, at the end of the modelling, both seismic and gravity constraints were respected with a sufficient fit between measured and calculated gravity data.

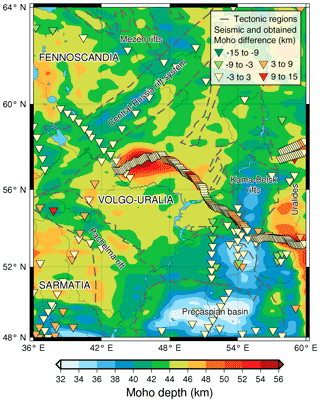
Figure 11 Moho model of Volgo–Uralian subcraton obtained through the gravity inversion with laterally variable density contrasts (Haas et al., 2020) and subsequent forward gravity modelling with seismic and gravity constraints in IGMAS+ (Götze and Lahmeyer, 1988; Schmidt et al., 2020). The comparison between the model which was obtained in the process of gravity inversion and the IGMAS+ Moho model is shown in Fig. S3 in the Supplement.

Most of the differences between seismic data and the Moho model developed in IGMAS+ are from the TATSEIS-2003 seismic profile. The seismic Moho depth along the TATSEIS-2003 and URSEIS-95 seismic profiles with respect to the model are shown in Fig. 12. As shown, within the TATSEIS profile seismic Moho has several steep troughs regarded as crustal roots (Artemieva and Thybo, 2013; Trofimov, 2006) which are not reflected in the satellite gravity field patterns. This case led us to a compromise solution: our Moho interface respects the main trends of Moho and at the same time smooths out its sharp gradients providing a closer fit to the gravity constraints. It can be seen that the Moho is deepest in the central cratonic region and it rises to the peripheral zone of Volgo–Uralia where it reaches the minimum depth of ca. 40 km under the thick sedimentary section of Sernovodsk–Abdulinsk Aulacogens. Then, the crustal–mantle interface drops sharply below 50 km after reaching the Uralides.

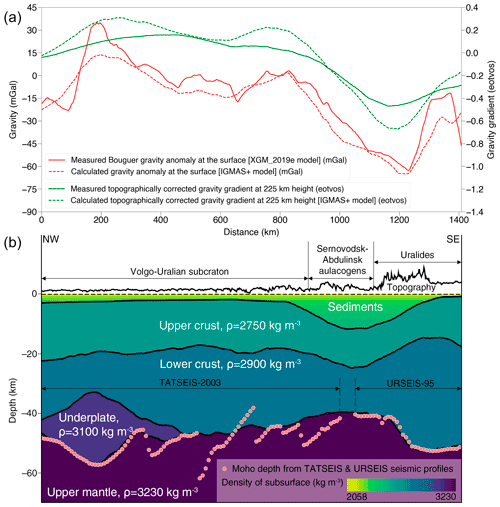
Figure 12 Measured and calculated Bouguer gravity anomalies and the topographically corrected vertical gravity gradient anomalies from the crustal model (a) and IGMAS+ model cross-section along TATSEIS-2003 and URSEIS-95 deep reflection profiles (b) – see Fig. 3 for the reference on the map. Subsurface is vertically exaggerated by the factor of 10 and topography is by the factor of 100.

A similar trend can be observed when looking at the north–south intersection of the eastern border of Volgo–Uralia (Fig. 13). The crust is the thickest in the northern cratonic region which is away from the palaeorifts. Whereas under the rift-like structures and in the cratonic area adjacent to them, the crust is thinning down. Particularly thin crust is observed in the south of the section under the Precaspian sedimentary basin.

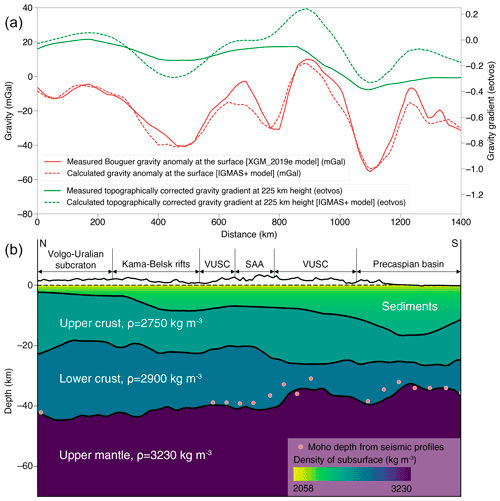
Figure 13 Measured and calculated Bouguer gravity anomalies and the topographically corrected vertical gravity gradient anomalies from the crustal model (a) and IGMAS+ model north–south cross-section in the eastern part of Volgo–Uralia (b) – see Fig. 3 for the reference on the map. Subsurface is vertically exaggerated by the factor of 10 and topography is by the factor of 100. VUSC stands for Volgo–Uralian subcraton and SAA for Sernovodsk–Abdulinsk Aulacogens.

The IGMAS+ model showed crustal thickness variation from 32 to more than 55 km in some areas. The thinnest crust with thickness below 40 km appeared on the Precaspian basin and Pre-Urals foredeep, which corresponds to the thickest sedimentary columns. A relatively thin crust was also found along the Central Russia rift system as well as in the north and south parts of the Pachelma rift. In the axial parts of both rifts, the thickness of the crust shrinks down to 40–42 km, while on the surrounding territory, the crust gains thickness back up to 44–46 km. Thick crust is located underneath the Ural Mountains as well as in the centre of the Volgo–Uralian subcraton. In each domain, crustal thickness exceeds 50 km. Overall, the developed model shows that within the EEC the Archaean cratonic blocks are related to the thickening of the crust and Palaeoproterozoic rifts are related to its thinning.

4.3 Comparison of the developed model to other regional Moho models

The resulting Moho model developed in IGMAS+ was cross-compared with the existing global and regional models which cover the studied region. For the comparison, the CRUST 1.0 global model (Laske et al., 2013), gravity-based GEMMA global model (Reguzzoni and Sampietro, 2015), and regional seismic EUNAseis model (Artemieva and Thybo, 2013) were selected. The difference between our model and the ones mentioned above is given in Fig. 14. It is clearly seen that the presented model is much deeper than GEMMA, and has more similar depths to the CRUST 1.0 and EUNAseis models. This is explained by the fact that our model as well as the CRUST 1.0 and especially seismic-only EUNAseis model are better constrained by the available seismic observations compared to the gravity-based GEMMA model.

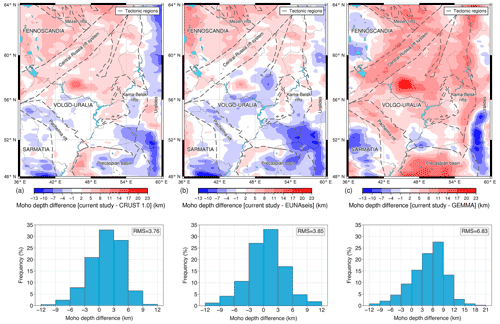
Figure 14 Difference in Moho depths between (a) obtained model developed in IGMAS+ and CRUST 1.0 model by Laske et al. (2013), (b) EUNAseis model by Artemieva and Thybo (2013), and (c) GEMMA model by Reguzzoni and Sampietro (2015). The top panel shows the maps of Moho depth residuals calculated as depth to Moho of the current study minus depth to Moho from the selected models in km. The bottom panel shows histograms of Moho depths differences in km.

When comparing our model to EUNAseis and CRUST 1.0, it becomes obvious that the obtained model is relatively deeper on the north-western part of the territory, which corresponds to Fennoscandia. One possible explanation for this feature is that the south-western part of Fennoscandia has relatively sparse coverage with seismic stations. This could have led to the discrepancy of the Moho depth on this zone estimated by gravity and seismic-based methods. As a result, the model developed during this study and GEMMA gravity-based model show 5–10 km deeper Moho for south-western Fennoscandia compared to CRUST 1.0 and EUNAseis.

Another significant difference that is seen between our model and CRUST 1.0 is the thicker crust in the centre of Volgo–Uralia in our model where the underplated body is recovered. Most probably, this difference has been revealed because the most recent seismic investigations on the Russian platform including the TATSEIS profile were not used in the compilation of CRUST 1.0. One can see that the EUNAseis model which has an extensive seismic database for the Russian platform is closer to our model in the centre of Volgo–Uralia where the underplated body is located.

The last conspicuous feature worth mentioning is the shallower Moho of the obtained model on the south-east of Volgo–Uralia as opposed to the EUNAseis model. Such anomaly arises because USGS seismic catalogue and EUNAseis seismic database have been built independently and have certain differences in seismic Moho estimations in this region. Our model respects more the seismic estimates of Moho depth given by the USGS catalogue on the south-east of Volgo–Uralia (Fig. 11) but diverges from EUNAseis Moho estimations showing 3–9 km shallower Moho in the south-eastern part of Volgo–Uralia and south of Ural Mountains.

5 Conclusions

We presented a new crustal model of the Volgo–Uralian subcraton obtained through gravity inversion and thorough forward gravity modelling with seismic constraints.

The gravity inversion was performed using laterally variable crust–mantle density contrasts. Three different density contrasts were estimated: 350 kg m⁻³ for the Precaspian sedimentary basin, 500 kg m⁻³ for Palaeoproterozoic rifts, and 550 kg m⁻³ for Archaean cratons and Uralides. The reference Moho depth was 45 km. As a result, we retrieved a gravity-inverted Moho depth of Volgo–Uralia. The gravity-inverted Moho model already exposed the major patterns of the crustal thickness variation in the area and was used as a preliminary layer in further 3D modelling.

Gravity field inversion was followed by 3D forward gravity modelling performed in IGMAS+ software. Here, additionally to gravity-inverted Moho, sedimentary, crustal, upper mantle, and asthenospheric layers were included in the model. Seismic estimates of the Moho depth, as well as the Bouguer gravity anomalies from the XGM2019e gravity field model and topographically corrected GOCE gravity gradient served as the main constraints for the modelling. The 3D forward gravity modelling revealed a considerable gravity misfit in the central part of the study area. We interpreted this misfit as an underplated body which is supported by the isostatic calculations. This reinforces the hypotheses of an underplated body located on the top of the Moho beneath the Oka block of Volgo–Uralia (Thybo and Artemieva, 2013).

The final crustal model respects all the main geological features of the Volgo–Uralian subcraton and its surroundings with Moho thickening in the cratons and under the Ural Mountains and thinning along the Palaeoproterozoic rifts, Precaspian sedimentary basin, and Pre-Urals foredeep. The obtained crustal model will serve as a basis for further basin analysis and geothermal modelling.