Can anaerobic oxidation of methane prevent seafloor gas escape in a warming climate?

Assessments of future climate-warming-induced seafloor methane (CH₄) release rarely include anaerobic oxidation of methane (AOM) within the sediments. Considering that more than 90 % of the CH₄ produced in ocean sediments today is consumed by AOM, this may result in substantial overestimations of future seafloor CH₄ release. Here, we integrate a fully coupled AOM module with a numerical hydrate model to investigate under what conditions rapid release of CH₄ can bypass AOM and result in significant fluxes to the ocean and atmosphere. We run a number of different model simulations for different permeabilities and maximum AOM rates. In all simulations, a future climate warming scenario is simulated by imposing a linear seafloor temperature increase of 3 ^∘C over the first 100 years. The results presented in this study should be seen as a first step towards understanding AOM dynamics in relation to climate change and hydrate dissociation. Although the model is somewhat poorly constrained, our results indicate that vertical CH₄ migration through hydraulic fractures can result in low AOM efficiencies. Fracture flow is the predicted mode of methane transport under warming-induced dissociation of hydrates on upper continental slopes. Therefore, in a future climate warming scenario, AOM might not significantly reduce methane release from marine sediments.

1 Introduction

The atmospheric concentration of CH₄ increased by a factor of 2.5 since the pre-industrial era, and anthropogenic emissions now account for 50 %–65 % of annual global CH₄ emissions (Stocker et al., 2013). CH₄ is an important greenhouse gas accounting for 20 % of the observed postindustrial climate warming (Kirschke et al., 2013). Marine sediments along continental margins contain large reservoirs of CH₄ stored as solid gas hydrate (Milkov, 2004; Wallmann et al., 2012). The stability of submarine CH₄ hydrate is primarily a function of temperature and pressure at and beneath the seafloor. Natural hydrate deposits are therefore susceptible to destabilization via ocean warming (Archer et al., 2009; Kretschmer et al., 2015; Dickens et al., 1995). The observed increase in atmospheric CH₄ content is presently attributed mostly to anthropogenic land use. However, a warming climate can lead to destabilization of the part of the marine hydrate reservoir sensitive to temperature perturbations, potentially leading to CH₄ transport from sediments to the oceans and atmosphere, where the CH₄ becomes a positive feedback on climate warming. As a result, anthropogenic-induced destabilization of natural marine CH₄ hydrate has been proposed as a climate warming mechanism that could exhibit threshold behaviour, implying that if climate warming continues, this feedback could cause an abrupt and irreversible transition into a warmer climate state (Stocker et al., 2013).

Although estimates of future CH₄ gas release to the atmosphere from hydrate destabilization on regional and global scales vary by orders of magnitude (Biastoch et al., 2011; Hunter et al., 2013; Kretschmer et al., 2015) and are likely overestimated (Stranne et al., 2016b), the Fifth Assessment Report of the Intergovernmental Panel on Climate Change evaluated the risk of a catastrophic CH₄ release during the 21st century as very unlikely (Stocker et al., 2013). In part, this is because much of the CH₄ escaping from the seafloor will be consumed in the water column before reaching the atmosphere (Mau et al., 2007; McGinnis et al., 2006). On longer timescales, however, the effect of widespread hydrate dissociation on our climate may be irreversible. This is due to the difference between timescales for release (discharge) and accumulation (recharge) – the recovery timescale from the perturbed state is significantly longer than the time it takes for the system to reach this perturbed state (Dickens, 2001; Kennett et al., 2003).

A mechanism that has been largely overlooked in this context, however, is anaerobic oxidation of methane (AOM) in marine sediments (Ruppel and Kessler, 2017). About 85 % of the annual global CH₄ production and 60 % of its consumption are based on microbial processes, and in marine sediments AOM is the dominant biogeochemical CH₄ sink (Egger et al., 2018; Knittel and Boetius, 2009; Martens and Berner, 1977; Reeburgh, 2007). AOM is carried out by microbes within the sulfate reduction zone (SRZ), a feature found in all anoxic marine sediments where the transport of methane from below and sulfate from above provides a source of energy through AOM (Barnes and Goldberg, 1976; Knittel and Boetius, 2009; Malinverno and Pohlman, 2011). It is estimated that, on a global scale, more than 90 % of the CH₄ produced in ocean sediments is consumed by AOM (Hinrichs and Boetius, 2002; Reeburgh, 2007). AOM is therefore a critical process that needs to be considered when modelling future climate-warming-induced CH₄ release from marine sediments.

Numerical methods for predicting future ocean warming-induced methane release from the marine hydrate reservoir span a wide range of complexities, from the simplest approaches where gas escape from the seafloor is estimated as a function of temperature change (Biastoch et al., 2011; Hunter et al., 2013; Kretschmer et al., 2015) to more sophisticated models that include coupled hydraulic–thermodynamic behaviour of multiphase fluid flow in hydrate-bearing porous media (Darnell and Flemings, 2015; Reagan et al., 2011; Reagan and Moridis, 2008; Stranne et al., 2016a; Thatcher et al., 2013). One example of the latter is the TOUGH+HYDRATE (T+H) model which predicts the evolution of pressure, temperature, salinity and the phase saturation distributions in hydrate-bearing systems (Moridis, 2014). Stranne et al. (2017) integrated a geomechanical coupling into the T+H model (referred to as T+H-GeoMech in the text) and showed that such coupling is critical since dissociation of methane increases pore pressure and leads to hydraulic fracturing. Hydraulic fractures increase the permeability of sediments and dramatically affect rates of dissociation and seafloor gas release. As the majority of the global marine methane hydrate reservoir is dominated by low-permeability, fine-grained (silt and clay) sediments (Boswell and Collett, 2011), hydraulic fracturing is an important mechanism controlling potential rates of methane release induced by climate warming. However, as pointed out by Ruppel and Kessler (2017), AOM in marine sediments is yet another important process that is missing in current numerical hydrate models.

In a warming world, AOM is the main mechanism that can potentially prevent the transfer of huge quantities of methane from sediments to the oceans. The efficiency of AOM under climate warming is still, however, a poorly constrained issue (Knittel and Boetius, 2009; Ruppel and Kessler, 2017). Although AOM efficiently controls the methane flux from the world's seafloors in general (Egger et al., 2018; Knittel and Boetius, 2009; Martens and Berner, 1977; Reeburgh, 2007), there are observational and model-based studies (Luff and Wallmann, 2003; Martens and Val Klump, 1980) suggesting that the rate of vertical CH₄ migration controls the efficiency of AOM (also referred to as the microbial filter). Buffett and Archer (2004) speculate that slow diffusive transport of CH₄ likely results in AOM within the sediments with negligible effect on climate, while a more rapid liberation of CH₄ (in response to climate warming) can lead to fractured pathways within the sediment that bypass the microbial filter and allow for a larger proportion of the CH₄ to reach the ocean and atmosphere. This idea is supported by Stranne et al. (2017), who showed that warming-induced hydrate dissociation in moderate- to low-permeability sediments (clays and silty clays) leads to formation of hydraulic fractures and rapid release of CH₄ from the seafloor.

In a review paper by Knittel and Boetius (2009), they list the following as one of the key future issues: “How will global climate change, with regard to the expected increase in temperature and sea level, affect the stability of gas hydrate reservoirs and the efficiency of microbial methane consumption?”. In a more recent review paper on the interaction between climate change and CH₄ hydrates (Ruppel and Kessler, 2017), the authors identify the quantification of the AOM sink in marine sediment as one of the key directions for future research. While Ruppel and Kessler (2017) recommend the use of numerical hydrate models for improved predictions of future warming-induced seafloor CH₄ release, they explicitly stress the need for better handling of AOM in such modelling efforts.

The present study aims at taking a step in this direction, through the addition of a simplistic but novel and fully coupled AOM module to the T+H-GeoMech code. As in Stranne et al. (2017), we focus on the featheredge of hydrate stability – the part of the marine hydrate reservoir most sensitive to ocean warming (Ruppel, 2011). We address the hypothesis of Buffett and Archer (2004) by investigating how the efficiency of the microbial filter varies as a function of the intrinsic permeability of the sediment (which in turn controls the vertical migration of CH₄) during seafloor warming-induced hydrate dissociation. In other words, to what extent can vigorous CH₄ flow through dynamic hydraulic fractures bypass the microbial filter? In all model simulations, a future climate warming scenario is simulated by imposing a linear seafloor temperature increase of 3 ^∘C over the first 100 years (Table 1).

2 Method

2.1 Model setup

T+H-GeoMech (Moridis, 2014; Stranne et al., 2017) is set up for midlatitude conditions with an initial bottom water temperature of 5 ^∘C and a gas hydrate stability zone (GHSZ) extending down to 20 m below the seafloor (m b.s.f.). This represents the most sensitive featheredge of hydrate stability on the upper continental slope. The initial hydrate deposit is homogeneously distributed within the GHSZ and is in thermodynamic equilibrium with the initial seafloor temperature, geothermal heat flow and the sediment bulk thermal conductivity profile. The model domain extends to 200 m b.s.f. and consists of 160 grid cells with a size of 0.17 m between 0 and 25 m b.s.f. and 19 m between 25 and 200 m b.s.f. We assume that the upper 5 m of the sediment column is within the SRZ and is initially depleted of CH₄ (Bhatnagar et al., 2011). See Table 1 for a list of parameter values used in the model simulations.

Table 1 Physical properties and T+H-GeoMech simulation parameters (for additional information, see Stranne et al., 2017).

^a From Thatcher et al. (2013). ^b From Stranne et al. (2017).

2.2 AOM module

The total vertically integrated CH₄ mass within the model domain is distributed between three pools (Fig. 1a): the hydrate pool (M_Hyd(t)), the gas pool (M_Gas(t)) and the dissolved pool (M_Dis(t)). CH₄ can move between these pools over time (t) and leave the system either through AOM within the SRZ (F_AOM(t,z)), where z is depth below seafloor, or through gas/dissolved CH₄ flux at the seafloor–ocean interface (F_Gas(t)∕F_Dis(t)). The F_AOM(t,z) is described as a sink on M_Dis(t), which means that gaseous CH₄ is not directly available for AOM. However, because pore water tends to be fully saturated in the presence of gas, AOM does act as a sink on M_Gas(t), as the constant reduction in CH₄ pore water saturation draws CH₄ from M_Gas(t) to M_Dis(t).

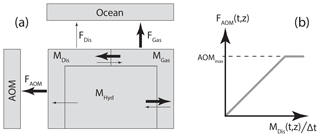
Figure 1 (a) A schematic overview of the three CH₄ mass pools within the sediments and the general direction of the CH₄ mass transport during hydrate dissociation, within and out of the system (illustrated by the thick arrows). (b) Modelled F_AOM(t,z) as a function of the dissolved CH₄ saturation (where Δt is the time step) and predefined AOM_max.

Observed AOM rates span from $\sim {\mathrm{10}}^{-\mathrm{6}}$ µmol cm⁻³ d⁻¹ in subsurface SRZs of deep margins to a few µmol cm⁻³ d⁻¹ in surface sediments above gas hydrates (Knittel and Boetius, 2009). In this study, we cover the range of maximum bulk oxidation rates within the SRZ (AOM_max) from zero to 1 µmol cm⁻³ d⁻¹ in Cases A1–A7 (Table 2, Fig. 2), within a predefined and constant depth of the SRZ extending, in the base case, to 5 m b.s.f. In each time step, the maximum amount of AOM is calculated (AOM_max multiplied by the time step and grid cell volume) in all grid cells within the SRZ. If the dissolved CH₄ content within a grid cell is smaller than or equal to the maximum amount of AOM, the dissolved CH₄ content is set to zero. The AOM within that particular grid cell is then limited by the dissolved CH₄ saturation. If the dissolved CH₄ content within a grid cell is larger than the maximum AOM, then the dissolved CH₄ content is reduced by this amount. The AOM within that grid cell is then limited by the predefined maximum AOM capacity of the system. This means that the modelled AOM rate is a linear function of dissolved CH₄ content (which is ultimately controlled by the CH₄ supply from below) up to a point where the predefined AOM_max takes over (Fig. 1b). In each grid cell where AOM occurs, an equal mass of water is added in order to keep mass balance within the system (i.e. CH₄ and NaCl are the only two dissolved species in the model, and therefore the end products from AOM are added to the water fraction of the pore space).

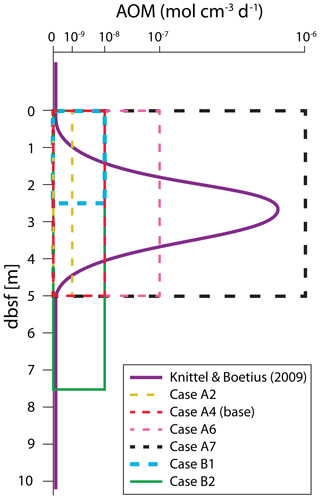
Figure 2 Conceptual AOM rate as a function of depth below seafloor (b.s.f., solid purple) based on Knittel and Boetius (2009) and a visual representation of some of the model simulation cases performed in the present study. In Cases A1–A7, the base of the SRZ is prescribed at 5 m b.s.f. We simulate two cases (B1–B2) with the SRZ extending down to 2.5 and 7.5 m b.s.f., respectively, both with an AOM_max of 10⁻⁸ mol cm⁻³ d⁻¹. Note that the x axis is non-linear and that all boxes (each representing a simulation case) have their upper left corner situated at the origin.

Table 2 Summary of the simulation cases performed in the present study. Each case involves 13 200-year simulations for permeabilities ranging between 10⁻¹⁷ and 10⁻¹⁴ m² (in total 143 simulations).

The base of the SRZ may be found at decimetres to tens of metres below the seafloor, depending on the burial rate of reactive organic matter, the depth of the methane production zone, the flux of methane and sulfate and their consumption rates (Egger et al., 2018; Knittel and Boetius, 2009). Our constant SRZ depth of 5 m b.s.f. represents a value commonly used in numerical modelling applied to marine gas hydrates (Kretschmer et al., 2015; Reagan and Moridis, 2008; Stranne et al., 2016a; Wallmann et al., 2012) and measured in high CH₄ flux areas at the featheredge (Miller et al., 2015; Rodrigues et al., 2017). Rodrigues et al. (2017) measured SRZ depths between 3 and 4 m b.s.f. in areas with high gas flow and approximately 7 m b.s.f. in background areas. We perform a sensitivity test on SRZ depth by running two additional suites of simulations with SRZ depth equal to 2.5 and 7.5 m in Cases B1–B2, respectively (Fig. S1 in the Supplement). The initial hydrate saturation (expressed as the percentage of pore space, S_h) in the baseline simulations is 5 %, homogeneously distributed within the GHSZ (except for the SRZ, which is initially depleted of hydrate). We perform a sensitivity test on the hydrate saturation by running two suites of simulations with S_h equal to 2.5 % and 7.5 % in Cases C1–C2, respectively (Fig. S2).

The efficiency of the microbial filter is defined as

$$\begin{array}{}\text{(1)}& {\displaystyle }\begin{array}{rl}& \mathrm{AOM}\mathrm{efficiency}=\\ & \left(\mathrm{1}-{\displaystyle \frac{{\left(F_{\mathrm{Gas}}\left(t\right)+F_{\mathrm{Dis}}\left(t\right)\right)}_{\mathrm{Case}\mathrm{A}x}}{{\left(F_{\mathrm{Gas}}\left(t\right)+F_{\mathrm{Dis}}\left(t\right)\right)}_{\mathrm{Case}\mathrm{A}\mathrm{1}}}}\right)\cdot \mathrm{100},\end{array}\end{array}$$

where Case Ax is one of the cases listed in Table 2. In other words, AOM efficiency is the percentage of CH₄ escape in the “zero AOM” case (Case A1) that is instead oxidized within the SRZ.

3 Results

As shown in Stranne et al. (2017), the upward transport of CH₄ within destabilized hydrate-bearing sediments can be divided into three flow regimes. These flow regimes depend on the sediment permeability and encompass the expected range of permeabilities for hemipelagic sediments composed predominantly of terrigenous silts and clays (Fig. 3).

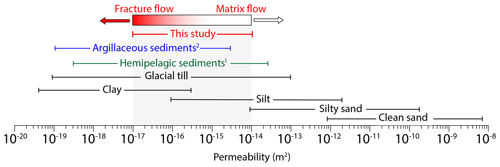
Figure 3 Typical range of permeability for unconsolidated sediments and marine sediments. Data sources are as follows: black – Freeze and Cherry (1979); green – Spinelli et al. (2004), porosity–permeability marine data compilation for porosities between 40 % and 85 %; blue – Neuzil (1994). Data from Neuzil (1994) are a compilation of laboratory permeability data for natural clay, silt sand mixtures from marine and terrestrial sources with porosities of 40 %–90 %.

The low-permeability fracture flow regime ($k<{\mathrm{10}}^{-\mathrm{15.5}}$ m²) is dominated by highly non-linear flow with irregular bursts of gas occurring at the seafloor through the opening and closing of hydraulic fractures (see Stranne et al., 2017, for details). When considering a centennial timescale, fracture flow results in the largest vertical transport of CH₄ gas towards the seafloor. In the matrix flow regime, which is predicted in higher-permeability substrate ($k>{\mathrm{10}}^{-\mathrm{15}}$ m²), CH₄ percolates through the porous media in a continuous, regular fashion through intergranular pore spaces. This slower flow regime will continue long after the hydrate deposit has been depleted because overpressure persists within the sediments and continues to drive vertical flow. This is distinct from fracture flow that ends the moment hydrate dissociation stops, because excess pore pressure no longer builds up within the sediments to create hydraulic fractures. These two regimes are separated by a mid-permeability low-flow regime (10${}^{-\mathrm{15.5}}\le k\le {\mathrm{10}}^{-\mathrm{15}}$ m²) where the permeability is high enough to allow gas transport away from the dissociation front (limiting the build-up of excess pore pressure and the formation of hydraulic fractures), while at the same time being low enough so that only small amounts of CH₄ reach the near-seafloor sediments on a centennial timescale. The development of the sediment column in terms of hydrate saturation, GHSZ, aqueous saturation and gas saturation for two model simulations with different permeabilities (Case A4) is shown in Fig. 4b–g. Seafloor CH₄ release as a function of time for the three fluid flow regimes is shown in Fig. 5a, d and g. Note that we use the terms “CH₄ escape” and “CH₄ gas escape” interchangeably throughout the text, as the dissolved fraction of the seafloor CH₄ escape is negligible (Figs. 5, 6c).

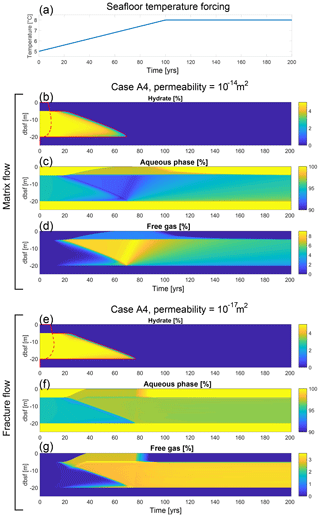
Figure 4 (a) Model simulations are all forced by a linear seafloor temperature increase of 3 ^∘C over the first 100 years. Modelled hydrate saturation (b, e), aqueous saturation (c, f) and gas saturation (d, g) as a function of time and depth b.s.f. for two simulations (Case A4) with permeability of 10⁻¹⁴ m² (b–d) and 10⁻¹⁷ m² (e–g), respectively. Also shown in panels (b) and (e) is the GHSZ, where the solid red line indicates the boundary for unstable conditions and the dashed red line indicates the boundary for stable conditions (area in between is at the melting point). The pressure development and the phase transition boundary at different times are shown in Fig. S3.

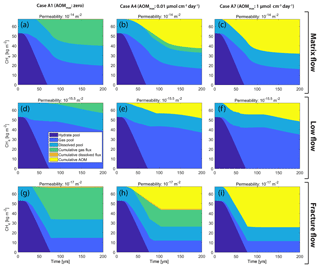
Figure 5 CH₄ mass budget over time showing the five components of Fig. 1 with the dissolved and gaseous fluxes separated. Displayed are three examples (high, mid- and low permeability; rows) for three different cases (Cases A1, A4 and A7; columns). Note that difference in cumulative CH₄ gas escape (green area) between high and low permeability is significantly larger in Case A4 (b, h) compared to Case A1 (a, g).

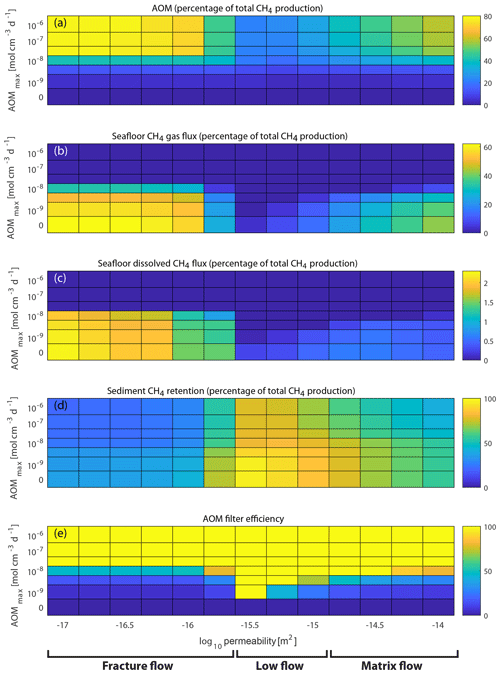
Figure 6 Case A1–A7 simulation results after 100 years (each tile represents one model simulation). Panels (a)–(d) show percentages of the total CH₄ production from hydrate dissociation after 100 years, which is identical in all cases and equal to 53 kg m⁻² (the sum of panels a–d equals 100 %). (a) The total cumulative AOM increases with increased AOM_max rates but is also a function of the vertical CH₄ flow rate within the sediments (highest values for the fractured flow regime, lowest values for the low-flow regime and intermediate values for the matrix flow regime). (b, c) The cumulative CH₄ release (b, gaseous; c, dissolved) decreases with increased AOM_max and also reflects vertical CH₄ flow rates within the sediments (as discussed above). (d) Sediment CH₄ retention is weakly dependent on AOM_max (some of the CH₄ that would reside within the SRZ in the zero AOM case would instead be consumed by AOM) but generally reflects the vertical CH₄ flow rates. (e) The AOM filter efficiency is defined as the fraction of CH₄ escape reduction compared to the corresponding zero AOM case (Case A1). For cases with AOM_max larger than 10⁻⁸ cm⁻³ d⁻¹, the model predicts that the microbial filter is 100 % effective, regardless of permeability, meaning that no CH₄ can escape from the seafloor. For lower AOM_max rates, the picture is more complex.

While permeability and fracture dynamics control the supply of CH₄ to the SRZ, the fate of CH₄ that reaches the SRZ is determined by the AOM_max rate. A high AOM_max rate leads to complete oxidation of the CH₄ before it can escape from the seafloor, while a low AOM_max leads to a large fraction of the CH₄ bypassing the microbial filter and escaping into the ocean. However, for intermediate AOM_max rates (around 10⁻⁸ cm⁻³ d⁻¹; Case A4), the efficiency of the microbial filter becomes a function of permeability (or flow regime). For the low-permeability fracture flow regime, with large vertical transport of CH₄, AOM is limited by the prescribed AOM_max rate; thus, an increase in CH₄ supply to the SRZ does not result in increased AOM but larger CH₄ escaping from the seafloor. For the low-flow regime, the opposite is true – all the supplied CH₄ to the SRZ is oxidized and none escapes, meaning that AOM becomes a sole function of the CH₄ supply. The matrix flow regime is somewhere in between these two extremes, and thus AOM and gas release are both strong functions of the CH₄ supply into the SRZ from below.

3.1 Case A4 (base case)

The fate of CH₄ produced from hydrate dissociation in Case A4 is visualized in Fig. 5b, e and h (where CH₄ production equals the hydrate reduction, shown as the dark blue area) and in Fig. 7a, c and e. It should be noted that the total CH₄ production is identical in all cases and equal to the amount of CH₄ initially stored in the hydrate deposit.

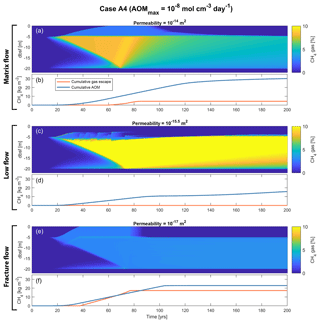
Figure 7 Example of simulation outputs from Case A4, highlighting the different dynamics of the three gas flow regimes within the sediments. Shown are sediment CH₄ gas saturation (percentage of pore space) with time and depth below the seafloor, and cumulative CH₄ gas escape and cumulative AOM with time, for three different permeabilities, representing higher-permeability matrix flow (a, b), mid-permeability low flow (c, d) and lower-permeability fracture flow (e, f). Note that the hydrate deposit is initially situated between 5 and 20 m b.s.f. and that gas is forming at the upper and lower edges of the deposit, which is gradually thinning and is completely dissociated after around 75 years into the simulations.

Figure 7 illustrates the radically different transport capacities of sediments with different permeability. In low-permeability sediments (Fig. 7e), fractures start to appear soon after the onset of hydrate dissociation (around 20 years into the simulation), effectively transporting most of the CH₄ gas away from the dissociation front and up towards the SRZ. The AOM capacity (as controlled by AOM_max) is smaller than the CH₄ supply, resulting in gas being released from the seafloor between 40 and 75 years into the simulation. Once the hydrate deposit is completely dissociated, fractures can no longer be created and the seafloor gas escape is immediately shut down. The remaining CH₄ within the SRZ is then consumed by AOM over the next 30 years (75 to 105 years into the simulation; Fig. 7f). The amount of CH₄ still residing within the sediment after 100 (200) years is about 24 % (22 %) of the produced CH₄ from hydrate dissociation (Fig. 6d). The transport of CH₄ in low-permeability sediments is mainly through fractures, which gives rise to variability of seafloor gas release on different timescales (Fig. 8a–c). This highly non-linear response to a constant seafloor warming is related to the opening and closing of fractures within the sediments, which occurs on timescales down to the order of hours (Fig. 8d).

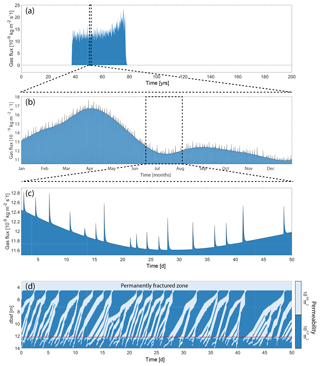
Figure 8 Fracture flow in low-permeability sediments for the base case simulation (Case A4 with permeability of 10⁻¹⁷ m²). Seafloor gas flux for the whole simulation (a), over 1 year (b) and over 50 d (c). (d) Fracture propagation within the sediments over the same period as panel (c). The horizontal red line marks the upper boundary of the hydrate deposit at this particular time, and the permanently fractured zone is the upper part of the sediments where the presence of gas alone is enough to create fractures (see Stranne et al., 2017, for details).

The CH₄ transport through high-permeability sediments is on average slower than in the low-permeability case, which is reflected by the higher CH₄ gas concentrations developing below the SRZ and by the gentler slope of the gas front rising up towards the seafloor with time (compare Fig. 7a with Fig. 7e). After about 60 years into the simulation, the vertical CH₄ transport finally overcomes the microbial filter and CH₄ gas starts to escape from the seafloor (Fig. 7b). The seafloor gas release continues for about 25 years (which is significantly shorter than the low-permeability gas release that continues for a period of about 40 years; Fig. 7f). After about 85 years into the simulation, the CH₄ supply to the SRZ is smaller than the AOM capacity (imposed by AOM_max), leading to a shutdown of seafloor CH₄ gas release and complete oxidation of any CH₄ that is transported into the SRZ. Due to the high permeability, gas continues to flow into the SRZ (although tapering off over time), where it is consumed by AOM. The amount of CH₄ retained within the sediments after 100 years is around 55 % (Fig. 6d). Sediments continue to oxidize CH₄, and after 200 years CH₄ retention is only about 35 %.

The CH₄ transport rate through mid-permeability sediments is significantly smaller than that through higher- and lower-permeability sediments, which is illustrated by the high CH₄ gas concentrations building up below the SRZ (Fig. 7c). Much of the CH₄ that ends up in the SRZ is mainly transported there through occasional fracturing. The CH₄ transport through fractures is not fast and large enough for any gaseous or dissolved CH₄ to escape the microbial filter, and the fraction of the produced CH₄ residing within the sediments is about 81 % (70 %) after 100 (200) years, which is significantly higher than the other cases (Fig. 6d).

The simulations in our base case (Case A4) show that, under some circumstances, sediment permeability and the associated flow dynamics control not only the transport of CH₄ from the dissociation front towards the seafloor (Stranne et al., 2017) but also the amount of CH₄ that escapes AOM within the SRZ. In Case A4, the efficiency of the microbial filter increases from about 45 % in low-permeability fracture flow-dominated sediments to 100 % in mid-permeability sediments and then decreases towards 80 % in high-permeability sediments (Fig. 6e). In absolute terms, this corresponds to a total CH₄ escape after 100 years of about 18 kg m⁻² in sediments with a permeability of 10⁻¹⁷ m² compared to a total CH₄ escape of around 4 kg m⁻² in sediments with a permeability of 10⁻¹⁴ m² – more than a factor of 4 difference, although part of the difference is associated with fluid flow dynamics within the sediments (Stranne et al., 2017).

4 Discussion

While AOM is important for understanding the potential impact of hydrate dissociation on climate across different timescales (Buffett and Archer, 2004), the strong AOM sink for CH₄ in marine sediments has not been previously assessed with numerical multiphase hydrate models (Ruppel and Kessler, 2017). In this study, we have integrated a simplistic but novel and fully coupled AOM module to the T+H-GeoMech code (Stranne et al., 2017) in order to investigate how AOM in marine sediments modify seafloor CH₄ release during dissociation of a marine hydrate deposit.

The results presented in Stranne et al. (2017) show that when naturally occurring marine hydrate deposits in low-permeability sediments (clay-dominated hemipelagic sediments; Fig. 3) are destabilized, transport of CH₄ towards the seafloor is facilitated by the formation of hydraulic fractures. This results in faster flow and ultimately larger fluxes of CH₄ compared to transport through higher-permeability sediments (silts and sands). Here, we show that, in addition, this type of fracture flow can circumvent the microbial filter more efficiently. The net effect can be substantial. In our base case (AOM${}_{max}={\mathrm{10}}^{-\mathrm{8}}$ mol cm⁻³ d⁻¹), the cumulative gas release after 100 years of seafloor warming is around 18 kg m⁻² in sediments with a permeability of 10⁻¹⁷ m², zero in sediments with a permeability of 10⁻¹⁵ m² and about 4 kg m⁻² in sediments with a permeability of 10⁻¹⁴ m² (Fig. 5b, e and h). This is in line with previous speculations (Archer et al., 2009; Buffett and Archer, 2004).

With an imposed upper limit of the AOM rate within the SRZ of around 10⁻⁸ cm⁻³ d⁻¹, the model can reproduce the observed relation between AOM efficiency and vertical CH₄ transport (Boetius and Wenzhöfer, 2013; Martens and Val Klump, 1980). For higher AOM capacities (AOM${}_{max}>{\mathrm{10}}^{-\mathrm{8}}$ cm⁻³ d⁻¹), AOM is sole function of the supply of CH₄ from beneath, and no gas escapes from the sediments. For lower capacities (AOM${}_{max}<{\mathrm{10}}^{-\mathrm{8}}$ cm⁻³ d⁻¹), on the other hand, the microbial filter efficiency is only marginal.

The efficiency of the microbial filter at some deep-sea cold seeps has been found to be rather limited (down to ∼20 %, Boetius and Wenzhöfer, 2013). In order to get such low efficiency in our simulations, the maximum bulk AOM rate (AOM_max) has to be lower than 10⁻⁸ mol cm⁻³ d⁻¹ (Fig. 6e). This is lower than what is often observed in these geological settings using experimental radiotracer-based methods (Niemann et al., 2006; Treude et al., 2003). There are at least two plausible explanations for this apparent discrepancy (in addition to differences in the definition of AOM efficiency): (1) high rates of AOM up to $\sim {\mathrm{10}}^{-\mathrm{3}}$ mol cm⁻³ d⁻¹ are observed to be highly localized spanning often no more than a few decimetres in studied sediment cores (e.g. Dale et al., 2010), which means that the average bulk AOM rate integrated over the full SRZ depth might be significantly lower; (2) deep-sea cold seeps might be very different from those forming at the featheredge of hydrate stability under rapid seafloor warming. Present-day cold seep systems have often been active for longer periods of time, sometimes tens of thousands of years (Berndt et al., 2014; Wallmann et al., 2018), and CH₄ is likely transported through high-permeability channels known as subsurface gas chimneys (Giambalvo et al., 2000; Saffer, 2015; Suess, 2010) or faults (Nakajima et al., 2014) through the GHSZ. Such channelled flow from the deep geosphere allows for significantly larger CH₄ transport than that through dynamic hydraulic fracturing (as considered in this study) because high-permeability channels stay open regardless of the in situ pore pressure. From the relation between vertical transport of CH₄ and AOM efficiency as found in observations and also presented in this study, a larger CH₄ transport would then also lead to lower AOM efficiencies. During rapid anthropogenic warming-induced hydrate dissociation, however, such high-permeability channels might not exist at the featheredge of the gas hydrate stability zone. We speculate, therefore, that the resulting flow would not resemble present-day cold seeps where gas is transported through subsurface gas chimneys or faults but that it would be more similar to that simulated in the present study, with the gas being transported either through elastic and highly dynamic (opening and closing) fractures in low-permeability sediments or percolating through the porous media in higher-permeability sediments.

There are limitations to the modelling approach applied in this study, and the results should be seen as a first step towards understanding AOM dynamics in relation to climate change and hydrate dissociation. One important limitation is that the model code does not consider kinetics, i.e. the rate of biogeochemical reactions. This means that the true efficiency of the microbial filter might be lower than reported here. We model AOM as a linear function of the CH₄ supply, with an upper AOM limit imposed by the AOM_max parameter. In reality, microbial communities are dynamic and adapt not only to the supply of CH₄ from beneath but also to changes in salinity, temperature and sulfate fluxes (Michaelis et al., 2002; Nauhaus et al., 2007; Treude et al., 2003). Experimental studies show that, for instance, a temperature increase of only 2 ^∘C can increase anaerobic organic matter degradation by 40 % (Roussel et al., 2015). In diffusive systems, the AOM process has been shown to operate at the thermodynamic limit for cell metabolism (Hoehler and Alperin, 1996), whereas advective systems apparently deliver CH₄ in amounts that allow for abundant cell growth and the development of thick biofilms capable of very high AOM rates (up to 10⁻⁴ mol cm⁻³ d⁻¹ (Boetius et al., 2000; Nauhaus et al., 2007; Treude et al., 2003). This implies that, while AOM is a highly complex process, the AOM rate within marine sediments is, to a first order, controlled by the CH₄ supply which is consistent with our model assumptions (Fig. 1b). We do not know what a realistic value of the maximum bulk AOM capacity could be or what is controlling it, but we note that an AOM_max rate of 10⁻⁸ mol cm⁻³ d⁻¹ reproduces the observed relation between AOM efficiency and CH₄ transport, at least qualitatively. It is possible that with the inclusion of proper kinetics and additional controls on the AOM process, there would be no need to impose such limitation on the AOM capacity.

Because the largest proportion of the sediment column is anoxic, the most important CH₄ sink in marine sediments globally is AOM (Knittel and Boetius, 2009). As a general rule, AOM dominates the CH₄ consumption within the sediments, while aerobic oxidation of CH₄ (AeOM) dominates the CH₄ consumption within the water column (Reeburgh, 2007; Valentine, 2011). AeOM in the benthic layer can, however, also be an important CH₄ sink. It has been shown that at some contemporary cold seeps, AeOM dominates over AOM (Boetius and Wenzhöfer, 2013). In this study, we focus on AOM, but as the AOM module does not discriminate between different types of oxidation, the modelled bulk CH₄ oxidation within the SRZ can in a sense be regarded as including all methane oxidation in the presence of sulfate, which thereby extends methane oxidation up to the seafloor where in reality other electron acceptors such as oxygen may oxidize methane.

Another limitation of the present model is the assumption of a static SRZ depth. In reality, the SRZ depth is dynamic, with a tendency to increase with decreasing methane flux from below (Borowski et al., 1996; Sivan et al., 2007). As the capacity of the microbial filter to oxidize CH₄ that passes through the SRZ depends on the SRZ depth (Fig. S1b), this tendency could decrease the filter efficiency during rapid dissociation of marine hydrates. Overall, the limitations of our modelling approach (including the lack of kinetics and of a dynamic SRZ depth) suggests that the AOM efficiency reported here can be regarded as an upper limit.

5 Conclusions

In general, the modelling results show that the total mass of CH₄ consumed by AOM over time becomes a function of either (1) the supply of CH₄ to the SRZ – when the AOM capacity (imposed by AOM_max) is so high that all the CH₄ transported to the SRZ is consumed by AOM or (2) the imposed AOM capacity itself – when the capacity is so low that there is an oversupply of CH₄ to the SRZ, which then also leads to CH₄ escaping the seafloor. In our simulations, the first case is true when AOM${}_{max}>{\mathrm{10}}^{-\mathrm{8}}$ mol cm⁻³ d⁻¹ (efficiency of the microbial filter is 100 %), while the second case is true when AOM${}_{max}<{\mathrm{10}}^{-\mathrm{8}}$ mol cm⁻³ d⁻¹ (AOM is negligible and the CH₄ escape is controlled by the sediment permeability). For values of AOM_max in between, on the order of 10⁻⁸ mol cm⁻³ d⁻¹, the AOM efficiency is to a large extent controlled by fluid flow rates (or sediment permeability), which is in line with observations. For example, during low-permeability CH₄ flow through fractures, the AOM efficiency (45 %) is about half that of high-permeability matrix flow (>80 %). The combination of larger CH₄ transport and lower AOM efficiency in low-permeability sediments ($\sim {\mathrm{10}}^{-\mathrm{17}}$ m²) results in a seafloor CH₄ release that is more than a factor of 4 larger than in high-permeability sediments ($\sim {\mathrm{10}}^{-\mathrm{14}}$ m²).

Although AOM in marine sediments is rarely considered when assessing future climate-warming-induced seafloor CH₄ release, there is a wealth of articles suggesting that it represents an important component of the marine CH₄ cycle. In this study, we can mimic the observed tendency of decreased AOM efficiencies with increased vertical CH₄ transport by imposing a maximum AOM bulk rate within the SRZ of about 10⁻⁸ mol cm⁻³ d⁻¹. We find that the AOM efficiency during fracture-dominated flow is less than 50 %, and this is likely an overestimation due to limitations in the AOM parameterization. Fracture flow is the predicted mode of methane transport under warming-induced dissociation of hydrates on upper continental slopes, and thus, in a scenario with rapidly warming seafloors, more (and possibly significantly more) than half of the CH₄ can escape AOM within the sediments and reach the ocean/atmosphere. These initial results are admittedly poorly constrained and will hopefully be augmented in future studies where kinetics and additional controls on AOM can be implemented. However, because evidence of ongoing anthropogenic warming-induced hydrate dissociation is inconclusive (Ruppel and Kessler, 2017) and observational data are still scarce, we have to at least partly rely on numerical hydrate models for the time being.